Graphical Modelling in Simile : Discrete event based

Event-based modelling in Simile

A pure discrete-event-based model includes only event symbols (time series and derived/delayed) and states. An event symbol only has a value at the point in time at which it occurs. The value of a derived event occurrence is calculated by its equation, which can include the value of the triggering event occurrence.  A state has a rule for each event symbol that influences it. When an influencing event occurs, the state's new value is given by the corresponding rule, otherwise it stays the same.

A model that mixes system dynamics (continuous) and discrete-event based operation also includes limit event symbols and squirts. A limit event occurrence is triggered when its equation reaches its minimum or maximum value, allowing continuous processes to set off events. A squirt is a derived event which causes an instantaneous transfer of material between compartments, allowing events to affect continuous processes. State changes can also affect continuous processes, but only squirts can cause discontinuities in the values of compartments.

See also the 5th video in the Simile Tutorial Series.

	event	An instantaneous occurrence which can have a magnitude
	state	A quantity set by events according to rules
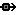	squirt	An instantaneous transfer of a substance between compartments
		If an influence originates from a discrete event valued component, it is shown 'bulged' to indicate that it carries a discrete event value. Such an influence cannot terminate on a continuous-valued component.
	immigration	The channel components which specify changes to the membership of population submodels can be either continuous or discrete valued. If one or more  discrete valued influence terminates on one of these, it is discrete valued and behaves like a squirt component, adding or removing as many submodel members immediately as the continuous version would over one time unit.
	reproduction
	removal

In: Contents >> Graphical Modelling